Answer:
(a) P(t) = 100 + 100(t²)
Bacteria grow at at exponential rate, which is what is being represented by the "t²" part of the expression. The number of cells initially present (100) also need to be added to the total population
(b) P(4) = 100 + 100(4²)
P(4) = 1700
replace 't' with '4' in the expression found in part (a) to calculate the number of bacterial cells after 4 hours
(c) P'(t) = 1700 + 1700(t²)
just plug in the size of bacterial population after 4 hours as has been calculated in the previous part of the question.
(d) 10000 = 100 + 100(t²)
here, we have the size of population given and need to find out the time it will take to get there, so make t the subject in the expression
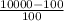 = t²
= t²
solve this expression to obtain the value of t²
t² = 99
take a square root on both sides to go from t² to t
t = 9.9 hours
Hence, in just 9.9 hours, the population of bacteria would have gone from being 100 initially to being 10,000!
Hope that answers the question. Have a great day!