Answer:
Using Sine rule we found the measure of ∠
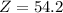 °.
°.
Explanation:
Diagram of the given condition shown below.
Given that,
In Triangle Δ
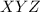 ,
,
∠
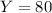 ° ,
° ,
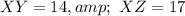 .
.
To find :- What is the measure of ∠
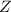 .
.
So,
Using Sine rule :- sine rule relates the sine of each angle to length of opposite side.

Where,
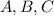 are angles of triangle Δ
are angles of triangle Δ
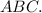
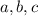 are side length of triangle.
are side length of triangle.
Then,
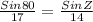
Using cross multiplication:-
⇒

⇒
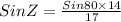
⇒
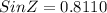
⇒ ∠
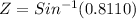
⇒ ∠
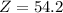 °
°
Hence,
Using Sine rule we found the measure of ∠
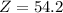 °.
°.