Question

Determine the equation of the polynomial, p(x),in both factored and standard form.

Solve the inequality p(x)<0.

Answer 1

We can see that the graph touches
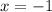`x=-1` without crossing the x-axis (i.e. it is a double solution), and then there's another zero at
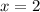`x=2` (this time it's a crossing zero, so a single solution).

This leads, up to multiple, to the polynomial

`p(x)=a(x+1)^2(x-2)`

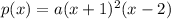
If we impose the passing through
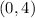`(0,4)` we have

`p(0)=4=a(1)(-2) \iff -2a=4 \iff a=-2`

So, the polynomial is

`p(x)=-2(x+1)^2(x-2)=-2 x^3 + 6 x + 4`

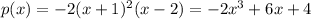
Finally, to solve
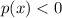`p(x)<0`, simply look at the graph, searching for the points, where the graph is below the x-axis. You can see that this happens only if
`x>2`, so that's the solution to your question.