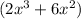Question

Charles begins finding the volume of a trapezoidal prism using the formula A = One-half(b1 + b2)h to find the prism's base area.

A = ((x + 4) + (x + 2))x
A = (2x + 6)x
A = (x + 3)x
A = x2 + 3x A trapezoidal prism is shown. The bases of the trapezoid have lengths of x + 2 and x + 4. The height of the trapezoid is x. The height of the prism is 2 x.
Which expression can be used to represent the volume of the trapezoidal prism?
2x3 + 6x2
x3 + 6x2
x3 + 3x2
2x3 + 3x2

Answer 1

(A) - 2x3 + 6x2

Explanation:

i got it right on edg 2020

Answer 2

The expression that can be used to represent the volume of the trapezoidal prism is
`(2x^3+6x^2)`

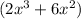
Explanation:

step 1

Find the area of the trapezoidal base

The area of a trapezoid is given by the formula

`A=(1)/(2)(b_1+b_2)h`

we have

`b_1=(x+2)\ units\\b_2=(x+4)\\h=x`

substitute

`A=(1)/(2)(x+2+x+4)x`

`A=(1)/(2)(2x+6)x`

`A=(x+3)x\\A=(x^2+3x)\ units^2`

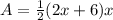
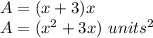
step 2

Find the volume of the trapezoidal prism

we know that

The volume of the prim is given by

`V=Bh`

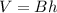
where

B is the area of the base

H is the height of the prism

we have

`B=(x^2+3x)\ units^2`

`H=2x\ units`

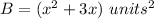
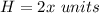
substitute

`V=(x^2+3x)2x\\V=(2x^3+6x^2)\ units^3`

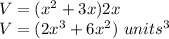
therefore

The expression that can be used to represent the volume of the trapezoidal prism is

`(2x^3+6x^2)`