Question

2NOCI (g)

→ 2NO(g) + Cl2 (g)
Problem:
Initially
the above reaction contains
0.50 M of NOCI,
0.00 M of NO,
0.00 M of C12.
Equilibrium Constant Kc = 1.6 (10^5)
What is the Equilibrium
Concentration of Cl2?

Answer 1

0.0097 mol·L⁻¹

Step-by-step explanation:

The balanced equation is

2NOCl ⇌ 2NO₂ + Cl₂

Data:

Your value of Kc is incorrect. It should be

Kc =1.6 × 10⁻⁵

[NOCl] = 0.50 mol·L⁻¹

[NO] = 0.00 mol·L⁻¹

[Cl₂] = 0.00 mol·L⁻¹

Calculations:

1. Set up an ICE table.

`\begin{array}{ccccccc}\rm \text{2NOCl}& \, \rightleftharpoons \, & \text{2NO} & +&\text{Cl}_(2) \\0.50 & &0.00 & & 0.00 & & \\-2x & & +2x & & +x & & \\0.50 -2x & & 0.00 + 2x & & 0.00 + x & & \\\end{array}`

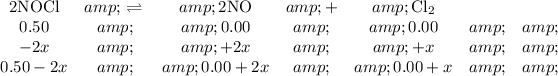
2. Calculate the equilibrium concentrations

`K_{\text{c}} = \frac{\text{[NO]$^(2)$[Cl$_(2)$]}}{\text{[NOCl]}^(2)} = ((2x)^(2)(x))/((0.50 - 2x)^(2)) = 1.6 * 10^(-5)\\\\4x^(3) = 1.6 * 10^(-5)(0.50 - 2x)^(2)\\x^(3) = 4.0 * 10^(-6)(0.50 - 2x)^(2)`

This is a cubic equation. Some calculators can solve cubic equations, but we can solve it by the method of successive approximations.

We will make changes to the right-hand side until the calculated value of x no longer changes,

(a) 1st approximation

Assume that 2x is negligible compared to 0.50. Then

`x = \sqrt [3] {4.0 * 10^(-6)(0.50)^(2)} = 0.010`

(b) 2nd approximation

Assume that x= 0.010. Then

`x = \sqrt [3] {4.0 * 10^(-6)(0.50 - 2* 0.010)^(2)} = 0.0097`

(b) 3rd approximation

Assume that x= 0.0097. Then

`x = \sqrt [3] {4.0 * 10^(-6)(0.50 - 2* 0.0097)^(2)} = 0.0097`

No change, so x = 0.0097.

[Cl₂] = x mol·L⁻¹ = 0.0097 mol·L⁻¹

Check:

`\begin{array}{rcl}((2* 0.0097)^(2) * 0.0097)/((0.050 - 2 *0.097)^(2)) & = & 1.6 * 10^(-5)\\\\(3.76 * 10^(-4) * 0.0097)/((0.050 - 0.0194)^(2)) & = & 1.6 * 10^(-5)\\\\(3.65 * 10^(-6))/((0.481)^(2)) & = & 1.6 * 10^(-5)\\\\(3.65 * 10^(-6))/(0.231) & = & 1.6 * 10^(-5)\\\\1.6* 10^(-5) & = & 1.6 * 10^(-5)\\\end{array}`

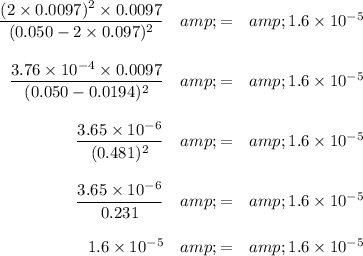
It checks.