Question

Find the critical points, domain endpoints, and local extreme values for the function

y=x^2/5(x+3)

a. What is/are the critical point(s) and domain endpoint(s) where f' is undefined?
b. What is/are the critical point(s) and domain endpoint(s) where f' is 0?
c. From the critical point(s) and domain endpoint(s), what is/are the points corresponding to local maxima?
d. From the critical point(s) and domain endpoint(s), what is/are the points corresponding to local minima?

Answer 1

a)
`x = -3`, b)
`x = 0`,
`x = -6`, c)
`x = 0`, d)
`x = -6`

Explanation:

a) Let derive the function:

`f'(x) = (10\cdot x \cdot (x+3)-5\cdot x^(2))/(25\cdot (x+3)^(2))`

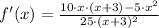
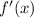`f'(x)` is undefined when denominator equates to zero. The critical point is:

`x = -3`

b)
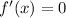`f'(x) = 0` when numerator equates to zero. That is:

`10\cdot x \cdot (x+3) - 5\cdot x^(2) = 0`

`10\cdot x^(2)+30\cdot x -5\cdot x^(2) = 0`

`5\cdot x^(2) + 30\cdot x = 0`

`5\cdot x \cdot (x+6) = 0`

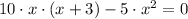
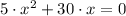
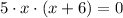
This equation shows two critical points:

`x = 0`,
`x = -6`

c) The critical points found in point b) and the existence of a discontinuity in point a) lead to the conclusion of the existence local minima and maxima. By plotting the function, it is evident that
`x = 0` corresponds to a local maximum. (See Attachment)

d) By plotting the function, it is evident that
`x = -6` corresponds to a local minimum. (See Attachment)