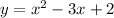Question

PLEASE HELP!!!!! I am giving 15 points

Write the quadratic equation in a standard form that represents the table below:

X Values are: -2, -1, 0, 1 and Y Values are: 12, 6, 2, 0

f(x)=

Answer 1

Explanation:

I used 3 of the coordinates to solve for the a, b, and c that we need in

`y=ax^2+bx+c`

We have 3 unknowns so we need 3 equations. The coordinates I used are from your table:

(-2, 12), (-1, 6), (0, 2). Start with (0, 2). Sub in a 2 for y and a 0 for x in the standard form of the quadratic:

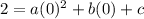`2=a(0)^2+b(0)+c` which simplifies very nicely to

c = 2. We will use that value now when we do this again using (-2, 12):

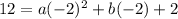`12=a(-2)^2+b(-2)+2` and

12 = 4a - 2b + 2 and

4a - 2b = 10 (1)

Do it again with the third coordinate (-1, 6):

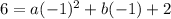`6=a(-1)^2+b(-1)+2` and

6 = 1a - b + 2 and

a - b = 4 (2)

Now we have a system of equations, (1) and (2) that we will combine and solve for a:

4a - 2b = 10

a - b = 4

Multiply the second equation by -2 to get a new system:

4a - 2b = 10

-2a + 2b = -8

Add to get

2a = 2 so

a = 1

Now sub in 1 for a in (2):

1 - b = 4 and

-b = 3 so

b = -3

Now we have all that we need to write the equation:

`y=x^2-3x+2`