Question

The probability of a train arriving on time and leaving on time is 0.8. The probability that the train arrives on time and leaves on time in 0.24. What is the probability that the train arrives on time, given that it leaves on time?

Answer 1

0.9524

Explanation:

Note enough information is given in this problem. I will do a similar problem like this. The problem is:

The Probability of a train arriving on time and leaving on time is 0.8.The probability of the same train arriving on time is 0.84. The probability of the same train leaving on time is 0.86.Given the train arrived on time, what is the probability it will leave on time?

Solution:

This is conditional probability.

Given:

• Probability train arrive on time and leave on time = 0.8
• Probability train arrive on time = 0.84
• Probability train leave on time = 0.86

Now, according to conditional probability formula, we can write:

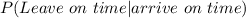`P(Leave \ on \ time | arrive \ on \ time)` = P(arrive ∩ leave) / P(arrive)

Arrive ∩ leave means probability of arriving AND leaving on time, that is given as "0.8"

and

P(arrive) means probability arriving on time given as 0.84, so:

0.8/0.84 = 0.9524

This is the answer.