Question

In the diagram, HK is tangent to Circle O. If HK is 24mm and the length from H to the

edge of the circle along OH is 18mm, what is the length of the radius of the circle?

Answer 1

The length of the radius of the circle is 7 mm

Explanation:

see the attached figure to better understand the problem

we know that

If HK is tangent to circle O at point K

then

The radius OK is perpendicular to segment HK and triangle OKH is a right triangle

Applying the Pythagorean Theorem

`OH^2=OK^2+HK^2`

we have

`OH=(r+18)\ mm\\OK=r\ mm\\HK=24\ mm`

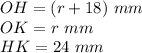
substitute

`(r+18)^2=r^2+24^2`

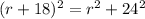
solve for r

`r^2+36r+324=r^2+576`

`36r=576-324\\36r=252\\r=7\ mm`

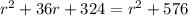
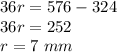
therefore

The length of the radius of the circle is 7 mm