Answer:
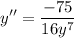
General Formulas and Concepts:
Calculus
Differentiation
- Derivatives
- Derivative Notation
Derivative Property [Multiplied Constant]:
![\displaystyle (d)/(dx) [cf(x)] = c \cdot f'(x)](https://img.qammunity.org/2021/formulas/mathematics/college/bz16ipe6p14y3f6abzxt2zy0j41tg530u9.png)
Derivative Property [Addition/Subtraction]:
![\displaystyle (d)/(dx)[f(x) + g(x)] = (d)/(dx)[f(x)] + (d)/(dx)[g(x)]](https://img.qammunity.org/2021/formulas/mathematics/college/kqosumt4896r7x44jgtw0o7kk6g4d3irvr.png)
Basic Power Rule:
- f(x) = cxⁿ
- f’(x) = c·nxⁿ⁻¹
Derivative Rule [Chain Rule]:
![\displaystyle (d)/(dx)[f(g(x))] =f'(g(x)) \cdot g'(x)](https://img.qammunity.org/2021/formulas/mathematics/college/ljowxevzhh8dk8mfdheam579ywk5jvteyi.png)
Implicit Differentiation
Explanation:
Step 1: Define
Identify

Step 2: Find 1st Derivative
- Differentiate [Basic Power Rule, Chain Rule, Derivative Properties]:
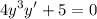
- Isolate y' term:
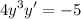
- Isolate y':
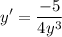
Step 3: Find 2nd Derivative
- Rewrite:

- Differentiation [Basic Power Rule, Chain Rule, Derivative Properties]:
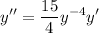
- Rewrite:

- Substitute in y':
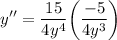
- Simplify:
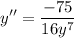
Topic: AP Calculus AB/BC (Calculus I/I + II)
Unit: Differentiation