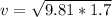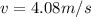Answer:
The linear velocity is
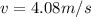
Step-by-step explanation:
According to the law of conservation of energy
The potential energy possessed by the hoop at the top of the inclined plane is converted to the kinetic energy at the foot of the inclined plane
The kinetic energy can be mathematically represented as

Where
 is the moment of inertia possessed by the hoop which is mathematically represented as
is the moment of inertia possessed by the hoop which is mathematically represented as
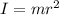
Here R is the radius of the hoop
 is the angular velocity which the hoop has at the bottom of the lower part of the inclined plane which is mathematically represented as
is the angular velocity which the hoop has at the bottom of the lower part of the inclined plane which is mathematically represented as
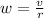
Where v linear speed of the hoop's center of mass just as the hoop leaves the incline and rolls onto a horizontal surface
Now expressing the above statement mathematically
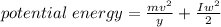
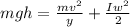
=>
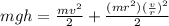
=>
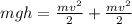
=>
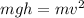
=>
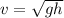
Substituting values