Answer:
μ = 0.423
Step-by-step explanation:
To solve this exercise we must use Newton's second law and kinematics together, let's start using expressions of kinematics to find the acceleration of the body
Let's fix a reference system where the x axis is parallel to the inclined plane, but the acceleration is only on this axis
x = v₀ t + ½ a t²
The body starts from rest so its initial speed is zero
a = 2 x / t²
a = 2 0.5 /0.5²
a = 4 m / s²
Taking the acceleration of the body, we use Newton's second law, we take the direction up the plane as positive
X axis
fr - Wₓ = m a (1)
Y Axis
N-
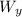 = 0
= 0
N = W_{y}
We use trigonometry to find the components of the weight
sin 45 = Wₓ / W
cos 45 = W_{y} / W
Wₓ = W sin 45
W_{y} = W cos 45
The out of touch has the expression
fr = μ N
fr = μ W_{y}
We substitute in 1
μ mg cos 45 - mg sin 45 = m a
W_{y} = (a + g sin 45) / g cos 45
μ = a / g cos 45 + 1
We calculate
Acceleration goes down the plane, so it is negative
a = -4 m / s²
μ = 1- 4 / (9.8 cos 45)
μ = 0.423