Question

An object at rest on a flat, horizontal surface explodes into two fragments, one seven times as massive as the other. The heavier fragment slides 9.10 m before stopping. How far does the lighter fragment slide?

Answer 1

445.9 m

Step-by-step explanation:

The law of conservation posits that the total momentum of two objects prior to collision is equal to the total momentum of the two objects after the collision has occurred.

i.e
`P_(initial) = P_(final)`

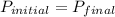
where;

`P = mv`

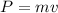
m = mass of the object

v = velocity of the object

here, the initial momentum
`P_(initial) = 0`

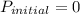
The final momentum
`P_(final)` = sum of the momentum of heavier fragment and lighter fragment.

`P_(final)` =
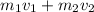`m_1v_1 +m_2v_2` ----- expression (1)

where

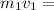`m_1v_1 =` the mass and the velocity of the lighter fragment

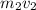`m_2v_2` = the mass and the velocity of the heavier fragment

From the question; we can say:

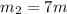`m_2 = 7m` (i.e the one that is seven times as massive as the other)

we write the above expression (1) as:

`P_(final) = mv_1 +7mv_2`

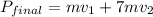
Since:

`P_(initial) = P_(final)`

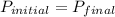
0 =
`P_(final)`

0 =
`mv_1 +7mv_2`

`- mv_1 = 7mv_2`

`v_1 = 7v_2`

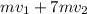
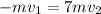
Let's determine the kinetic energy and the work done by the frictional force in both fragments

To start with the lighter fragment; the kinetic energy is expressed as;

`\delta \ K.E = W_f\\(1)/(2) mv^2 = \mu mg \delta x`

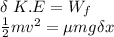
where;

m = mass

v = v₁ = velocity

`\delta x = \delta x_1` = change in direction

So;

`(1)/(2)mv_1^2 = \mu mg \delta x_1`

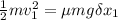
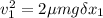`v_1^2 = 2 \mu mg \delta x_1` --------- equation (1)

For the heavier fragment ;

`\delta \ K.E = W_f\\(1)/(2) mv_2^2 = \mu mg \delta x_2`

`(1)/(2) 7mv_2^2 = \mu (7m)g (9.10m)`

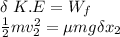
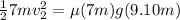
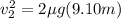`v_2^2 = 2 \mu g (9.10m)` ------------ equation (2)

Equating equation (1) by equation (2); we have:

`(v_1^2)/(v_2^2)= (2 \mu g \delta x_1)/(2 \mu g (9.10 m))`

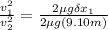
Replacing
`v_1 = 7v_2` ; from the above relation of the velocities; we have

`((7v_2)/(v_2))^2 = (\delta x_1)/((9.10\ m))`

`(49)/(1) * (\delta x_1)/((9.10 \ m))`

`\delta x_1 = 49 * 9.10 \ m \\ \delta x_1 = 445.9 \ m`

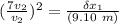
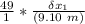
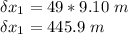
∴ The lighter fragment slide 445.9 m far from the initial point of explosion.

Answer 2

Answer: 445.9 m

Step-by-step explanation:

Since momentum is conserved, the lighter fragment then has a velocity seven times the heavier fragment.

Also, the force if friction will be seven times less than the force of friction in the heavier fragment.

Initial kinetic energy of the lighter fragment is seven times that of the heavier fragment.

This then mean, the force would need 49 times a larger distance to perform the work of stopping.

49 * 9.1 = 445.9 m