Question

g The force that a 60 Kg block applies to an ideal spring (initially in its equilibrium position) produces a work 90 J , and stretches the spring 0.1 cm . How much work is required to stretch the spring by 1 cm from its equilibrium position

Answer 1

W = 9 kJ

Step-by-step explanation:

We have:

m: mass of the block = 60 kg

W: work = 90 J

x: distance = 0.1 cm = 1.00x10⁻³ m

To find the work we can use the following equation:

`W = F*x = (1)/(2)kx^(2)`

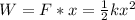
Where:

F: is the force that the block applies to an ideal spring = -kx

First, we need to find the spring constant:

`k = (2W)/(x^(2)) = (2*90 J)/((1.00 \cdot 10^(-3) m)^(2)) = 1.80 \cdot 10^(8) N/m`

Now, with the spring constant we can find the work required to stretch the spring by 1 cm from its equilibrium position:

`W = (1)/(2)kx^(2) = (1)/(2)1.80 \cdot 10^(8) N/m*(1.00 \cdot 10^(-2) m)^(2) = 9000 J = 9 kJ`

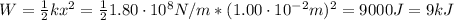
Therefore, the work required is 9 kJ.

I hope it helps you!

Answer 2

Work = 9000J

Step-by-step explanation:

Work done on the spring is 60J

mass of block 60kg

extension on the spring 0.1cm

Workdone =
`(1)/(2)`Ke²

where K is the force constant of the spring, Inputting the values

we have 90 =
`(1)/(2)`×K×0.1²

K =
`(180)/(0.01)`

K = 18000 N/cm

How much work is required to stretch the spring by 1 cm from its equilibrium position, we have

Workdone =
`(1)/(2)`Ke²

work =
`(1)/(2)`×18000×1²

Work = 9000J

= 9KJ