Question

A 10 KgKg wheel that is 40 cmcm in diameter rotates through an angle of 19 radrad as it slows down uniformly from 16 rad/secrad/sec to 26 rad/secrad/sec . What is the magnitud of the angular acceleration of the wheel?

Answer 1

The magnitude of angular acceleration of the wheel = 0.26
`(rad)/(s^(2) )`

Step-by-step explanation:

Mass m = 10 kg

Diameter = 0.4 m

Initial speed
`\omega_(1) = 26 \ (rad)/(sec)`

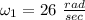
Final speed
`\omega_(2) = 16 \ (rad)/(sec)`

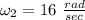
Angle
`\theta` = 19 rad

The value of final speed is given by

`\omega _(2) = \omega _(1) + 2 \alpha \theta`

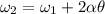
Put all the values in above equation we get

16 = 26 + 2 (
`\alpha`) (19)

`\alpha` = - 0.26
`(rad)/(s^(2) )`

Therefore the magnitude of angular acceleration of the wheel = 0.26
`(rad)/(s^(2) )`