Answer:
Probability that L is greater than 1.20 milligrams per liter is 0.5488.
Explanation:
We are given that based on data collected from metal shredders across the nation, the amount L of extractable lead in metal shredder residue has an approximate exponential distribution with mean μ = 2.0 milligrams per liter.
The probability distribution for exponential distribution is given by;
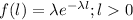
where,
 = parameter of this distribution
= parameter of this distribution
Let L = Amount of extractable lead in metal shredder residue
Now, as we know that the mean of exponential distribution is;
Mean,
 =
=
 ⇒ 2.0 =
⇒ 2.0 =
 {because we are given with the mean}
{because we are given with the mean}
So,
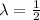 = 0.5
= 0.5
Hence, L ~ Exp(
 )
)
Now, to find the less than or greater than probabilities in exponential distribution we use the Cumulative distribution function of exponential function, i.e.;
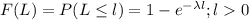
So, probability that L is greater than 1.20 milligrams per liter is given by = P(L > 1.20 milligrams per liter)
P(L > 1.20) = 1 - P(L
 1.20)
1.20)
= 1 - [
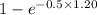 ]
]
=
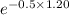 = 0.5488
= 0.5488
Therefore, probability that L is greater than 1.20 milligrams per liter is 0.5488.