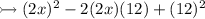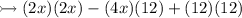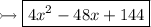Question

Expand and simplify (2x−12) ^2

Answer 1

`\boxed{4x^(2) - 48x + 144}`

Explanation:

Given expression:

• (2x - 12)²

To simplify the expression, we will use the formula (a - b)² = a² - 2ab + b².

[Where "a and b" are the first and second term in (a - b)²]

`\rightarrowtail (2x - 12)^(2)`

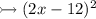
In this case, the first term of (2x - 12)² is "2x" and the second term is "12".

`\rightarrowtail (2x)^(2) - 2(2x)(12) + (12)^(2) \ \ \ \ \ \ \ \ \ \ \ \ \ \ [\small\text{First term = a = 2x; Second term = b = 12]}`

Now, simplify the expression.

`\rightarrowtail (2x)^(2) - 2(2x)(12) + (12)^(2)`

`\rightarrowtail (2x)(2x) - (4x)(12) + (12)(12)`

`\rightarrowtail \boxed{4x^(2) - 48x + 144}`