Answer:
(a) The probability that the randomly selected Rayovac battery will have a test life between 20.4 and 22.2 hours is 0.4178.
(b) The probability that the sample mean of 5 Rayovac battery will have a mean test life between 20.4 and 22.2 hours is 0.6104.
(c) The probability that the sample mean of 15 Rayovac battery will have a mean test life between 20.4 and 22.2 hours is 0.7019.
(d) The probability that the sample mean of 69 Rayovac battery will have a mean test life between 20.4 and 22.2 hours is 0.8708.
Explanation:
Let X = the life time of Rayovac batteries.
The lifetime of Rayovac batteries is Normally distributed with mean μ = 20.6 hours and standard deviation σ = 1.47 hours.
The standardized value of the sample mean of X is:
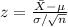
(a)
Compute the probability that the randomly selected Rayovac battery will have a test life between 20.4 and 22.2 hours as follows:

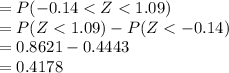
Thus, the probability that the randomly selected Rayovac battery will have a test life between 20.4 and 22.2 hours is 0.4178.
(b)
Compute the probability that the sample mean of 5 Rayovac battery will have a mean test life between 20.4 and 22.2 hours as follows:

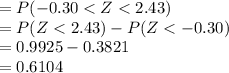
Thus, the probability that the sample mean of 5 Rayovac battery will have a mean test life between 20.4 and 22.2 hours is 0.6104.
(c)
Compute the probability that the sample mean of 15 Rayovac battery will have a mean test life between 20.4 and 22.2 hours as follows:
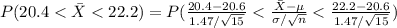

Thus, the probability that the sample mean of 15 Rayovac battery will have a mean test life between 20.4 and 22.2 hours is 0.7019.
(d)
Compute the probability that the sample mean of 69 Rayovac battery will have a mean test life between 20.4 and 22.2 hours as follows:
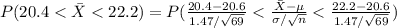
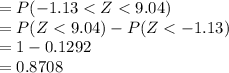
Thus, the probability that the sample mean of 69 Rayovac battery will have a mean test life between 20.4 and 22.2 hours is 0.8708.