Answer:
The speed of the car at the bottom of the circle is twice as its speed at the top of the circle.
Step-by-step explanation:
It is given that, at the bottom of the circle, the car experiences four times as much radial (centripetal) acceleration as at the top of the circle such that :
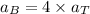
The centripetal acceleration is given by :
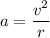
Let
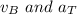 are the speed of the roller coaster car at bottom and at top respectively.
are the speed of the roller coaster car at bottom and at top respectively.
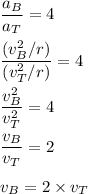 .
.
So, the speed of the car at the bottom of the circle is twice as its speed at the top of the circle. Hence, the correct option is (D).