Question

An insulated tank that contains 1 kh of O2 and 15C and 300 kPa is connected to a 2m uninsulated tank that contains N2 at 50C and 500 kPa. The valve connecting the two tanks is opened, and the two gases form a homogenous mixture at 25C. Determine the final pressure in the tank, the heat transfer?

Answer 1

The final pressure of the mixture = 444.4 k pa

Step-by-step explanation:

Given data for oxygen

Mass of oxygen = 1 kg

Temperature = 15 °c= 298 K

Pressure P = 300 K pa

Volume of the oxygen

`V = ((1)(0.257)(298))/(300)`

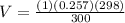
V = 0.25
`m^(3)`

Mole number of oxygen is

`N o_(2) = (m)/(M)`

`N o_(2) = (1)/(32)`

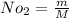
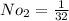
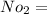`N o_(2) =` 0.03125 k mol

Given data for nitrogen

Volume of Nitrogen = 2
`m^(3)`

Temperature = 50 °c= 323 K

Pressure = 500 K pa

Mass of nitrogen

m =
`((500)(2))/((0.297)(323))`

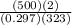
m = 10.43 kg

Now mole number of nitrogen

`N n_(2) = (10.43)/(28)`

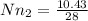
`N n_(2) =` 0.372 k mol

Thus the mole number of mixture is the sum of mole no. of oxygen & mole no. of nitrogen.

N =
`N o_(2) + N n_(2)`

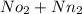
N = 0.03125 + 0.372

N = 0.4035 k mol

Therefore the final pressure of the mixture is given by the ideal gas equation

P V = N R T ------- (1)

Where P = final pressure of the mixture

V = 0.25 + 2 = 2.25
`m^(3)`

N = total no. of moles in final mixture = 0.4035 K mol

T = final temperature of the mixture = 298 K

Put all the values in equation 1 , we get

P × 2.25 = 0.4035 × 8.314 × 298

P = 444.4 K pa

Therefore the final pressure of the mixture = 444.4 k pa