Answer:
The estimated probability that between 60 and 63 children of the sample are from poverty households is 0.1367.
Explanation:
Let X = number of American children under the age of 6 live in households with incomes less than the official poverty level.
The probability of the random variable X occurring is, p = 0.22.
A random sample of children of size n = 300 is selected.
The random variable X follows a Binomial distribution with parameters n = 300 and p = 0.22.
A normal approximation to Binomial can be used to approximate the distribution of X if the following conditions are fulfilled:
Check:
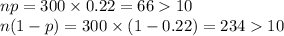
So, the random variable X approximately follows N (np, np(1 - p)).
Compute the probability of X between 60 and 63 as follows:

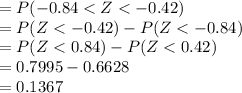
*Use a z-table for the probability values.
Thus, the estimated probability that between 60 and 63 children of the sample are from poverty households is 0.1367.