Answer: (a) Percentage of 25 year old men that are above 6 feet 2 inches is 11.5%.
(b) Percentage of 25 year old men in the 6 footer club that are above 6 feet 5 inches are 2.4%.
Explanation:
Given that,
Height (in inches) of a 25 year old man is a normal random variable with mean
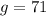 and variance
and variance
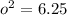 .
.
To find: (a) What percentage of 25 year old men are 6 feet, 2 inches tall
(b) What percentage of 25 year old men in the 6 footer club are over 6 feet. 5 inches.
Now,
(a) To calculate the percentage of men, we have to calculate the probability
P[Height of a 25 year old man is over 6 feet 2 inches]= P[X>
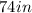 ]
]
P[X>74] = P[
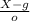 >
>
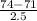 ]
]
= P[Z > 1.2]
= 1 - P[Z ≤ 1.2]
= 1 - Ф (1.2)
= 1 - 0.8849
= 0.1151
Thus, percentage of 25 year old men that are above 6 feet 2 inches is 11.5%.
(b) P[Height of 25 year old man is above 6 feet 5 inches gives that he is above 6 feet] = P[X,
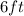
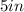 - X,
- X,
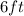 ]
]
P[X >
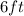
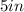 I X >
I X >
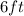 ] = P[X > 77 I X > 72]
] = P[X > 77 I X > 72]
=
![(P[X > 77])/(P[ X > 72])](https://img.qammunity.org/2021/formulas/mathematics/high-school/6vs085bb1d8pmb66r72gaw0tczppozo0xq.png)
=
![(P[(X - g)/(o)>(77-71)/(2.5)] )/(P[(X-g)/(o) >(72-71)/(2.5)] )](https://img.qammunity.org/2021/formulas/mathematics/high-school/vnathitxj1y2lx5k345wsbv3ouf0qxvskj.png)
=
![(P[Z >2.4])/(P[Z>0.4])](https://img.qammunity.org/2021/formulas/mathematics/high-school/6d4xvsemrnm9hqmwowgikd5xprbm97ny8o.png)
=
![(1-P[Z\leq2.4] )/(1-P[Z\leq0.4] )](https://img.qammunity.org/2021/formulas/mathematics/high-school/wdg6hxiudf2h625skpdo6af2s4ovqpxaa9.png)
=
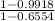
=
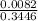
= 0.024
Thus, Percentage of 25 year old men in the 6 footer club that are above 6 feet 5 inches are 2.4%.