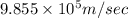Question

What is the velocity of a beam of electrons that goes undeflected when passing through perpendicular electric and magnetic fields of magnitude 6800 V/m and 6.9×10−3 T , respectively?

Answer 1

The velocity of a beam of electrons is
`9.85* 10^(-5)\ m/s`.

Step-by-step explanation:

Given that,

Electric field,
`E=6800\ V/m`

Magnetic field,
`B=6.9* 10^(-3)\ T`

When electric and magnetic field both act on the particle, the net force is given by :

`F=(qE+qv* B)`

If the electron moves undeflected, F = 0

So,

`E=v* B\\\\v=(E)/(B)\\\\v=(6800)/(6.9* 10^(-3))\\\\v=9.85* 10^5\ m/s`

So, the velocity of a beam of electrons is
`9.85* 10^(-5)\ m/s`. Hence, this is the required solution.

Answer 2

Velocity of electron will be equal to
`9.855* 10^5m/sec`

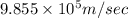
Step-by-step explanation:

We have given electric field is equal to E = 6800 volt/m

Magnetic field is equal to
`B=6.9* 10^(-3)T`

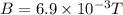
We have to find the velocity of the electron

We know that velocity of electron is equal to ratio of electric field and magnetic field

So
`v=(E)/(B)=(6800)/(6.9* 10^(-3))=9.855* 10^5m/sec`

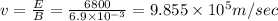
Velocity of electron will be equal to
`9.855* 10^5m/sec`