Answer:
23.97% probability that a customer has to wait more than 5 minutes.
Explanation:
The exponential probability distribution, with mean m, is described by the following equation:
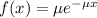
In which
 is the decay parameter.
is the decay parameter.
The probability that x is lower or equal to a is given by:
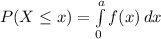
Which has the following solution:
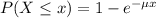
The manager of a fast-food restaurant determines that the average time that her customers wait for service is 3.5 minutes.
This means that
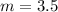 . So
. So
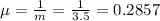
Find the probability that a customer has to wait more than 5 minutes.
Either the customer has to wait for 5 minutes or less, or he has to wait for more than 5 minutes. The sum of the probabilities of these events is decimal 1. So
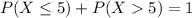
We want
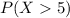 . So
. So

In which
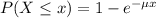
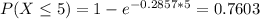
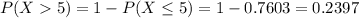
23.97% probability that a customer has to wait more than 5 minutes.