Answer:
a) By the Central Limit Theorem, the distribution would be approximately normal, with mean
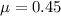 and standard deviation
and standard deviation
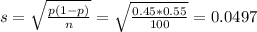
b) 4.02 standard deviations below the mean.
c) Making 25 or less shots has a pvalue of -4.02. Z-scores lower than -2 are considered surprising, so yes, it would be surprising for him to make only 25 of these shots.
Explanation:
To solve this question, we are going to need to understand the normal probability distribution and the central limit theorem.
Normal probability distribution
Problems of normally distributed samples are solved using the z-score formula.
In a set with mean
 and standard deviation
and standard deviation
 , the zscore of a measure X is given by:
, the zscore of a measure X is given by:

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.
Central Limit Theorem
The Central Limit Theorem estabilishes that, for a normally distributed random variable X, with mean
 and standard deviation
and standard deviation
 , the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean
, the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean
 and standard deviation
and standard deviation
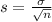 .
.
For a skewed variable, the Central Limit Theorem can also be applied, as long as n is at least 30.
For proportions, we have that the mean is
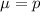 and the standard deviation is
and the standard deviation is

In this problem, we have that:
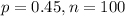
a)By the Central Limit Theorem, the distribution would be approximately normal, with mean
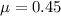 and standard deviation
and standard deviation
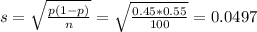
b)This is Z when X = 0.25.

By the Central Limit Theorem
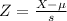
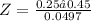

4.02 standard deviations below the mean.
c) Making 25 or less shots has a pvalue of -4.02. Z-scores lower than -2 are considered
surprising, so yes, it would be surprising for him to make only 25 of these shots.