Answer: The standard entropy change of the given reaction is -186.51
Step-by-step explanation:
Entropy change is defined as the difference in entropy of all the product and the reactants each multiplied with their respective number of moles.
The equation used to calculate entropy change is of a reaction is:
![\Delta S^o_(rxn)=\sum [n* \Delta S^o_((product))]-\sum [n* \Delta S^o_((reactant))]](https://img.qammunity.org/2021/formulas/chemistry/college/pjqszh23uhn70w1i5wjdf2c6tt6auxvc1t.png)
For the given chemical reaction:
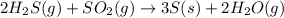
The equation for the entropy change of the above reaction is:
![\Delta S^o_(rxn)=[(3* \Delta S^o_((S(s))))+(2* \Delta S^o_((H_2O(g))))]-[(2* \Delta S^o_((H_2S(g))))+(1* \Delta S^o_((SO_2(g))))]](https://img.qammunity.org/2021/formulas/chemistry/college/upyqivuoi6hzkr40od4ty87vrq508z5xpz.png)
We are given:
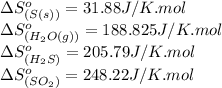
Putting values in above equation, we get:
![\Delta S^o_(rxn)=[(3* (31.88))+(2* (188.825))]-[(2* (205.79))+(1* (248.22))]\\\\\Delta S^o_(rxn)=-186.51J/K](https://img.qammunity.org/2021/formulas/chemistry/college/uqegmfi19n0c1eref57isu9xkh2ms7icfc.png)
Hence, the standard entropy change of the given reaction is -186.51