Question

In the laboratory a student combines 23.5 mL of a 0.173 M barium sulfide solution with 27.7 mL of a 0.402 M sodium sulfide solution. What is the final concentration of sulfide anion ?

Answer 1

Answer: The final concentration of sulfide anion in the solution is 0.297 M

Step-by-step explanation:

To calculate the number of moles for given molarity, we use the equation:

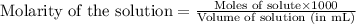`\text{Molarity of the solution}=\frac{\text{Moles of solute}* 1000}{\text{Volume of solution (in mL)}}` .....(1)

• For barium sulfide:

Molarity of barium sulfide solution = 0.173 M

Volume of solution = 23.5 mL

Putting values in equation 1, we get:

`0.173M=\frac{\text{Moles of barium sulfide}* 1000}{23.5}\\\\\text{Moles of barium sulfide}=(0.173* 23.5)/(1000)=0.0041mol`

1 mole of barium sulfide (BaS) produces 1 mole of barium ions
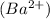`(Ba^(2+))` and 1 mole of sulfide ions
`(S^(2-))`

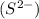
Moles of sulfide ions = (1 × 0.0041) = 0.0041 moles

• For sodium sulfide:

Molarity of sodium sulfide solution = 0.402 M

Volume of solution = 27.7 mL

Putting values in equation 1, we get:

`0.402M=\frac{\text{Moles of sodium sulfide}* 1000}{27.7}\\\\\text{Moles of sodium sulfide}=(0.402* 27.7)/(1000)=0.0111mol`

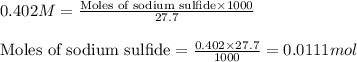
1 mole of sodium sulfide
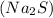`(Na_2S)` produces 2 moles of sodium ions
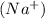`(Na^(+))` and 1 mole of sulfide ions
`(S^(2-))`

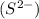
Moles of sulfide ions = (1 × 0.0111) = 0.0111 moles

Now, calculating the concentration of sulfide anion in the solution by using equation 1, we get:

Total moles of sulfide ions = [0.0041 + 0.0111] = 0.0152 moles

Total volume of the solution = [23.5 + 27.7] = 51.2 mL

Putting values in equation 1, we get:

`\text{Molarity of }S^(2-)\text{ ions}=(0.0152* 1000)/(51.2)\\\\\text{Molarity of }S^(2-)\text{ ions}=0.297M`

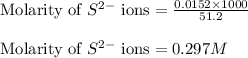
Hence, the final concentration of sulfide anion in the solution is 0.297 M