Question

A candy box is made from a piece of cardboard that measures 15 by 9 inches. Squares of equal size will be cut out of each corner. The sides will then be folded up to form a rectangular box. What size square should be cut from each corner to obtain maximum​ volume?

Answer 1

1.82 inches

Explanation:

Let the size of square piece cut from the corner is d.

So, the length of the box is 15 - 2y

width of the box is 9 - 2y

height of the box is d.

Volume of the box

Volume = length x width x height

V = (15 - 2y)(9 - 2y)y

V = 4y³ - 48y² + 135y

Differentiate with respect to y.

`(dV)/(dy)=12y^(2)-96y+135`

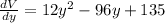
It is equal to zero for maxima and minima

`12y^(2)-96y+135=0`

`y=\frac{96\pm \sqrt{96^(2)-4* 12* 135}}{24}`

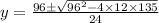
So, y = 1.82 in or 6.2 in

Now

`\frac{d^(2){V}}{dy^(2)}=24y-96`

For y = 1.82 in, it is negative

and for y = 6.2 in, it is positive

So, the volume of the box is maximum if the height of the box is 1.82 inch.

Thus, the size of the square cut from each of the corner is 1.82 inches.