Question

Consider the following balanced equation for the following reaction:

15O2(g) + 2C6H5COOH(aq) → 14CO2(g) + 6H2O(l)
Determine the amount of CO2(g) formed in the reaction if the percent yield of CO2(g) is 83.0% and the theoretical yield of CO2(g) is 1.30 moles.

Answer 1

Answer: The actual yield of the carbon dioxide is 47.48 grams

Step-by-step explanation:

For the given balanced equation:

`15O_2(g)+2C_6H_5COOH(aq.)\rightarrow 14CO_2(g)+6H_2O(l)`

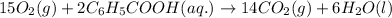
To calculate the mass for given number of moles, we use the equation:

`\text{Number of moles}=\frac{\text{Given mass}}{\text{Molar mass}}`

Theoretical moles of carbon dioxide = 1.30 moles

Molar mass of carbon dioxide = 44 g/mol

Putting values in above equation, we get:

`1.30mol=\frac{\text{Theoretical yield of carbon dioxide}}{44g/mol}\\\\\text{Theoretical yield of carbon dioxide}=(1.30mol* 44g/mol)=57.2g`

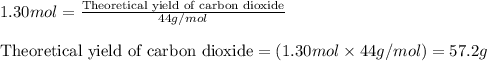
To calculate the theoretical yield of carbon dioxide, we use the equation:

`\%\text{ yield}=\frac{\text{Actual yield}}{\text{Theoretical yield}}* 100`

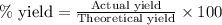
Theoretical yield of carbon dioxide = 57.2 g

Percentage yield of carbon dioxide = 83.0 %

Putting values in above equation, we get:

`83=\frac{\text{Actual yield of carbon dioxide}}{57.2}* 100\\\\\text{Actual yield of carbon dioxide}=(57.2* 83)/(100)=47.48g`

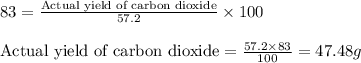
Hence, the actual yield of the carbon dioxide is 47.48 grams