Question

The journey of an electron through an external circuit involves a long and slow zigzag path that is characterized by losses in electric potential. We now look extensively at resistance - the cause for these voltage drops. Resistivity is defined by the ratio of the magnitude to electric field and current density. Resistance is related to resistivity by R= rhoL/A for cylindrical conductors. Two wires - A and B - with circular cross-sections have identical lengths and are made of the same material. Yet, wire A has four times the resistance of wire B. How many times greater is the diameter of wire B than wire A?

Answer 1

d_{b} = 2 d_{a}

Step-by-step explanation:

The electrical resistance for a cylindrical wire is described by the expression

R = ρ L / A

The area of ​​a circle is

A = π r²

r = d / 2

A = π d²/4

We substitute

R = ρ L 4 /π d²

Let's apply this expression to our case, they indicate that the resistance of wire A is 4 times the resistance of wire B

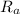`R_(a)` = 4 R_{b}

We substitute

ρ 4/π
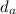`d_(a)`² = 4 (ρ 4/π d_{b}²)

1 / d_{a}² = 4 / d_{b}²

d_{a} = d_{b} / 2