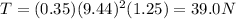Question

A student swings a 0.35 kg rock on a 1.25 m string above their head. The rock goes through 1 complete revolution in 0.665 s. What is the tension in the string?

Answer 1

39.0 N

Step-by-step explanation:

Since the rock is in uniform circular motion, the tension in the string provides the centripetal force required to keep the rock in circular motion.

So we can write:

`T=m\omega^2 r`

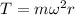
where

T is the tension in the string

m = 0.35 kg is the mass of the rock

r = 1.25 m is the radius of the circle (the length of the string)

`\omega` is the angular velocity of the rock

Here the rock covers 1 revolution in a time of

t = 0.665 s

1 revolution corresponds to an angle of
`2\pi` rad, so the angular velocity is:

`\omega=(2\pi)/(0.665 s)=9.44 rad/s`

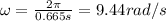
Therefore substituting into the previous equation, we can find the centripetal force, which is the tension in the string:

`T=(0.35)(9.44)^2(1.25)=39.0 N`