Answer:
Centripetal force doubles
Step-by-step explanation:
Since centripetal force is given by
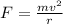
Where F is the centripetal force, m is the mass, v is the centripetal velocity and r is circle radius.
When the radius is reduced to half then the centripetal force will be
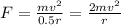
Evidently, the centripetal force is doubled compared to the initial.
Note that while the question gives letter v for radius, I used v for velocity and r for radius since these are the standard letters for abbreviating formula of centripetal force.