Answer:
The function is not continuous or rather say it is discontinuous at x = 1
Explanation:
For a function to be continuous at a specific value, it must satisfy the following requirement:
- f(a) exists and is finite (A function at specific value can be evaluated.)
- Both left and right limit are equivalent which leads to limit existing. (limit has to exist.)
With the two requirement, a function is continuous at specific interval or value.
We are given a function:
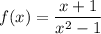
First, we factor the expression x²-1 using the difference of two squares.
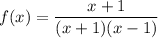
Cancel x+1.
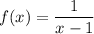
This is our new function where domain cannot be x = 1 since that will make a function undefined. With this information, we can conclude that the function is not continuous as it fails the f(a) exists and is finite. (Even without factoring the expression, it is obvious that the original function cannot he evaluated at x = -1 because that will also make a function undefined.)