Question

EMERGENCY (NEED HEP NOW!!!)

Solve the system of linear equations.

4x - y = -9

3x - 3y = 0

Answer 1

x=-2.25,y=-2.25

Explanation:

The given system is

`4x - y = - 9 \\ 3x - 3y = 0`

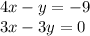
We want to solve the system for x and y that makes the system true.

Let us multiply the first equation by 3 while maintaining the second equation:

`12x -3 y = - 27 \\ 3x - 3y = 0`

Subtract the current second equation from the first one.

`12x = - 27`

Divide through by 12:

`x = - (27)/(12) = - 2.25`

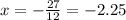
From the second equation we have:

`3x = 3y`

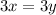
This implies that:

`x = y = - 2.25`