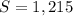Answer:
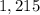
Explanation:
we have
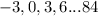

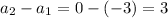
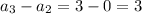

so
The common difference d is 3
we know that
The rule to find the sum of the the first n terms of the arithmetic sequence is equal to
![S=(n)/(2) [2a_1+(n-1)d]](https://img.qammunity.org/2021/formulas/mathematics/middle-school/40a9f2kh2yeqdlm2wspv7nie3t5wvn1omp.png)
where
d is the common difference
a_1 is the first term
we have
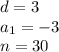
substitute in the formula
![S=(30)/(2) [2(-3)+(30-1)3]](https://img.qammunity.org/2021/formulas/mathematics/middle-school/5rr5218t2bc34omccpmxwhnbnx3ondssoj.png)
![S=15[-6+87]](https://img.qammunity.org/2021/formulas/mathematics/middle-school/44fnsm83542z78nby8k7i6oym6rdm4ftfb.png)