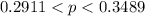Question

The latest poll from ESPN asked fans what their favorite sport was, considering pro, college and highschool levels. Out of 1000 fans, 32% of fans said their favorite sport was college basketball. Construct a 95% confidence interval.

Answer 1

Confidence interval is
`0.2911<p<0.3489`

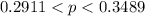
Explanation:

Given that 32% of fans sport is basketball as favorite sport. So total number of fans is,

`32\%* 1000`

`= (32)/(100)* 1000`

`=320`

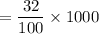
So 320 fans favorite sport is basket ball.

Now find sample proportion ,

`\widehat{p}=(number\:of\:people\:in\:the\:sample)/(total\:number\:of\:people)`

Substituting the value,

`\widehat{p}=(320)/(1000)`

`\widehat{p}=0.320`

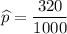
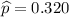
Formula for confidence interval is,

`CI=\widehat{p}\pm z\sqrt{\frac{\widehat{p}\left (1-\widehat{p}\right )}{n}}`

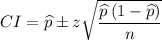
To calculate the z value.

`Confidence Level=1-\alpha`

`0.95=1-\alpha`

`0.05=\alpha`

Now divide above value by 2,

`(0.05)/(2)=(\alpha)/(2)`

`0.025=(\alpha)/(2)`

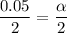
To find the value of z score calculate the area under the curve as follows

`A=(1+CL)/(2)`

`A=(1+0.95)/(2)`

`A=(1.95)/(2)`

`A=0.975`

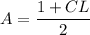
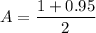
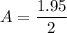
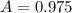
On z table, find the value of 0.975. So the row corresponding to 0.975 is 1.9 and column corresponding to 0.975 is 0.06. Adding these two values the z score is 1.96.

or find the value from excel by using command as follows,

=NORM.S.INV(0.025)= -1.959963985

Now substituting the value,

`CI=0.320\pm 1.96\sqrt{(0.320\left (1-0.320\right ))/(1000)}`

`CI=0.320\pm 0.02891`

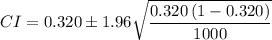
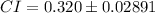
Lower end of the interval is,

`CI=0.320- 0.02891`

`CI=0.2911`

Upper end of the interval is,

`CI=0.320+ 0.02891`

`CI=0.3489`

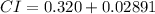
Therefore 95% confidence interval is
`0.2911<p<0.3489`