Given inequality:
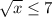
To find:
Which values are solutions to the inequality.
Solution:
Substitute the values in the x place.
Option A: 48
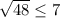
6.9 ≤ 7
Therefore 48 is one solution of the inequality.
It is true.
Option B: -5
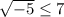
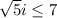
We cannot compare a complex number and a real number.
Therefore -5 is not a solution of the inequality.
it is not true.
Option C: 50
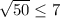
7.07 > 7
Therefore 50 is not a solution of the inequality.
It is not true.
Option D: 49
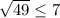
7 ≤ 7
Therefore 49 is one solution of the inequality.
It is true.
Option E: 44

6.6 ≤ 7
Therefore 44 is one solution of the inequality.
It is true.
Option F: 2401
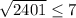
49 > 7
Therefore 2401 is not a solution of the inequality.
It is not true.
Therefore 48, 49 and 44 are solutions to the inequality.