I assume the integral is
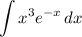
Integrate by parts with
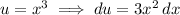
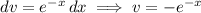
so that
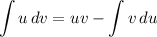
i.e.

Integrate by parts again, this time with
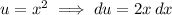
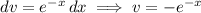
and so
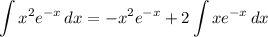
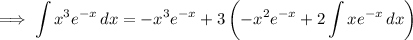

Once more, with
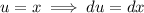
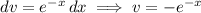
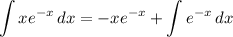
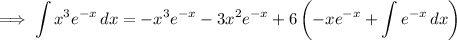

Finally,
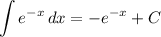
and so

or equivalently,
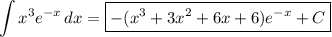
We can also approach the integral more generally by considering
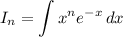
Integrate by parts with
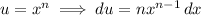
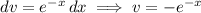
Then
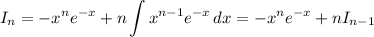
We can solve the recurrence using the initial "value"
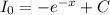 to find a general formula for
to find a general formula for
 . Then we get the integral we want
. Then we get the integral we want
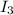 "for free".
"for free".