Question

A rectangular box with a volume of 684 ftcubed is to be constructed with a square base and top. The cost per square foot for the bottom is 20cents​, for the top is 10cents​, and for the sides is 2.5cents. What dimensions will minimize the​ cost?

Answer 1

The dimensions of the rectangular box is 29.08 ft×29.08 ft×4.85 ft.

Minimum cost= 26,779.77 cents.

Explanation:

Given that a rectangular box with a volume of 684 ft³.

The base and the top of the rectangular box is square in shape.

Let the length and width of the rectangular box be x.

[since the base is square in shape, length=width]

and the height of the rectangular box be h.

The volume of rectangular box is = Length ×width × height

=(x²h) ft³

According to the problem,

`x^2h=684`

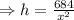`\Rightarrow h=(684)/(x^2)`.....(1)

The area of the base and top of rectangular box is = x² ft²

The surface area of the sides= 2(length+width) height

=2(x+x)h

=4xh ft²

The total cost to construct the rectangular box is

=[(x²×20)+(x²×10)+(4xh×2.5)] cents

=(20x²+10x²+10xh) cents

=(30x²+10xh) cents

Total cost= C(x).

C(x) is in cents.

∴C(x)=30x²+10xh

Putting
`h=(684)/(x^2)`

`C(x)=30x^2+10x*(684)/(x^2)`

`\Rightarrow C(x)=30x^2+(6840)/(x)`

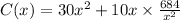
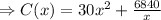
Differentiating with respect to x

`C'(x)=60x-(6840)/(x^2)`

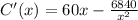
To find minimum cost, we set C'(x)=0

`\therefore60x-(6840)/(x^2)=0`

`\Rightarrow60x=(6840)/(x^2)`

`\Rightarrow x^3=(6840)/(60)`

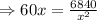
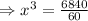
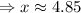`\Rightarrow x\approx 4.85` ft.

Putting the value x in equation (1) we get

`h=(684)/((4.85)^2)`

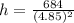
≈29.08 ft.

The dimensions of the rectangular box is 29.08 ft×29.08 ft×4.85 ft.

Minimum cost C(x)=[30(29.08)²+10(29.08)(4.85)] cents

=29,779.77 cents