Question

What is the energy of cell phone radiation with 1m wavelength

Answer 1

`1.99\cdot 10^(-25) J`

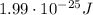
Step-by-step explanation:

Electromagnetic waves are oscillations of the electric and the magnetic field in a plane perpendicular to the direction of motion the wave.

All electromagnetic waves travel in a vacuum always at the same speed, the speed of light:

`c=3.0\cdot 10^8 m/s`

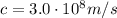
The energy of an electromagnetic wave is given by the equation:

`E=(hc)/(\lambda)`

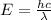
where:

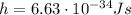`h=6.63\cdot 10^(-34)Js` is the Planck constant

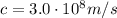`c=3.0\cdot 10^8 m/s` is the speed of light

`\lambda` is the wavelength of the wave

In this problem, we have a wave with wavelength of

`\lambda=1 m`

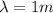
So, its energy is:

`E=((6.63\cdot 10^(-34))(3.0\cdot 10^8))/(1)=1.99\cdot 10^(-25) J`