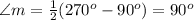Question

Circle A is shown. Tangents L M and N M intersect at point M outside of the circle. Arc L N is 270 degrees. In the diagram of circle A, what is m? 75° 90° 120° 135°

Answer 1

B

Explanation:

Answer 2

`\angle m=90^o`

Explanation:

The picture of the question in the attached figure

we know that

The measurement of the external angle is the half-difference of the arches that comprise

so

`\angle m=(1)/(2)(major\ arc\ LN-minor\ arc\ LN)`

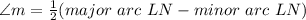
Remember that the sum of the major arc and a minor arc is equal to 360 degrees

we have

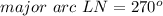`major\ arc\ LN=270^o` ----> is given

so

`minor\ arc\ LN=360^o-270^o=90^o`

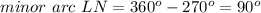
substitute the values

`\angle m=(1)/(2)(270^o-90^o)=90^o`