Answer:
Increase
Step-by-step explanation:
The period of an oscillating system is the time it takes for the system to complete 1 oscillation.
The period of a spring-mass oscillating system is given by
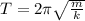
where
k is the spring constant
m is the mass
We see that:
- The period is proportional to the square root of the mass: so as the mass increases, the period increases too (1)
- The period is inversely proportional to the square root of the spring constant: so as the constant decreases, the period increases (2)
In this problem:
- The mass of the system is increased
- The spring constant is decreased
Therefore, according to observations (1) and (2), the period of the system will increase.