Answer:
(a) The factors are (x + 4), (10 - x) and (x - 70).
(b) There is a break-even for 70 units of the product.
Explanation:
(a)
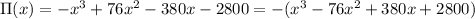
Factorizing the term in parentheses,
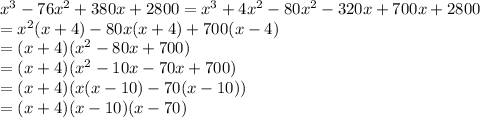
Then
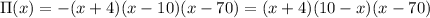
The factors are (x + 4), (10 - x) and (x - 70).
(b)
Break-even occurs when Π(x) = 0
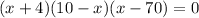
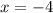 or
or
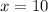 or
or
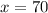
Since x cannot be negative, x = 70.
Hence, there is a break-even for 70 units of the product.