Given that the isosceles triangle has sides √45, √45 and x.
The triangle has a height of 3 units.
We need to determine the value of x.
Value of x:
The value of x can be determined using the formula,
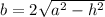
Where b is the base of the triangle, a is the sides of the isosceles triangle and h is the height of the triangle.
Substituting
 ,
,
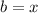 and
and
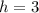 in the above formula, we get;
in the above formula, we get;
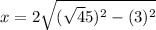
Squaring the terms, we have;
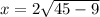
Subtracting, we get;
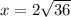
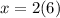
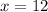
Thus, the value of x is 12
Hence, Option B is the correct answer.