the rate of change between 0 to 90 days is 2.74 i.e. 2.74 flies per day !
Explanation:
Here we have , Suppose that we began with 2 flies and after the 90 day (3 month) experiment, there were 248.000 flies. We need to find What is the rate of change between 0 to 90 days . Let's find out:
We know that for a function f(x) , rate of change of function over interval [a,b] :
⇒
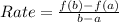
Applying the same concept here with following parameters as :
[a,b] = [0,90]
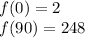
⇒
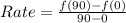
⇒

⇒
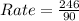
⇒
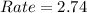
Therefore , the rate of change between 0 to 90 days is 2.74 i.e. 2.74 flies per day !