Answer:
Intensity = 11.56W/m²
The energy flowing through the given area is 4.55 J
Step-by-step explanation:
The expression for the intensity of the electromagnetic wave is,
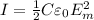
Here,
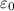 is the permittivity of the free space,
is the permittivity of the free space,
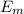 is the electric field amplitude and
is the electric field amplitude and
c is the speed of the light.
substitute
⁸m/s for c
8.85×10 −12 C² /N⋅m² for
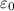
and 93.3 V/m for


The expression for the energy is,
E = I×A×t
Here, I is the intensity of the electromagnetic wave,
A is the area, and
t is the time.
Substitute
11.56W/m² for I
0.0287m ² for A
13.7s for t
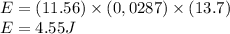
The energy flowing through the given area is 4.55 J