Question

Suppose that scores on a test are normally distributed with a mean of 80 and a standard deviation of 8. Which of the following questions below are of type B? a. Find the 80th percentile. b. Find the cutoff for the A grade if the top 10% get an A. c. Find the percentage scoring more than 90. d. Find the score that separates the bottom 30% from the top 70%. e. Find the probability that a randomly selected student will score more than 80.

Answer 1

a) 86.73

b) 90.24

c) 10.56% scoring more than 90

d) 75.8

e) 50% probability that a randomly selected student will score more than 80.

Explanation:

Problems of normally distributed samples can be solved using the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the zscore of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the pvalue, we get the probability that the value of the measure is greater than X.

In this problem, we have that:

`\mu = 80, \sigma = 8`

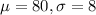
a. Find the 80th percentile.

This is the value of X when Z has a pvalue of 0.8. So X when Z = 0.841.

`Z = (X - \mu)/(\sigma)`

`0.841 = (X - 80)/(8)`

`X - 80 = 8*0.841`

`X = 86.73`

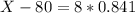
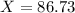
b. Find the cutoff for the A grade if the top 10% get an A.

This is the value of X when Z has a pvalue of 0.9. So X when Z = 1.28.

`Z = (X - \mu)/(\sigma)`

`1.28 = (X - 80)/(8)`

`X - 80 = 8*1.28`

`X = 90.24`

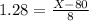
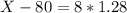
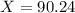
c. Find the percentage scoring more than 90.

This is 1 subtracted by the pvalue of Z when X = 90. So

`Z = (X - \mu)/(\sigma)`

`Z = (90 - 80)/(8)`

`Z = 1.25`

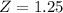
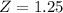`Z = 1.25` has a pvalue of 0.8944.

1 - 0.8944 = 0.1056

10.56% scoring more than 90

d. Find the score that separates the bottom 30% from the top 70%.

This is the value of X when Z has a pvalue of 0.3. So X when Z = -0.525.

`Z = (X - \mu)/(\sigma)`

`-0.525 = (X - 80)/(8)`

`X - 80 = 8*(-0.525)`

`X = 75.8`

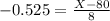
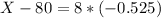
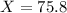
e. Find the probability that a randomly selected student will score more than 80.

This is 1 subtracted by the pvalue of Z when X = 80.

`Z = (X - \mu)/(\sigma)`

`Z = (80 - 80)/(8)`

`Z = 0`

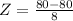
`Z = 0` has a pvalue of 0.5.

1 - 0.5 = 0.5

50% probability that a randomly selected student will score more than 80.