Answer:
No, Method is not effective.
Explanation:
Let x be the number of girls = 319
and n be the total number of babies = 580
Then, proportion
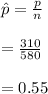
Standard Error,
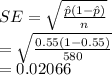
The level of significance is:

Confidence Interval:
![p \pm [Z_{(\alpha)/(2)} * SE]\\=0.550 \pm [2.575 * 0.02066\\=(0.4968, 0.6032)](https://img.qammunity.org/2021/formulas/mathematics/college/ssgfep8e8c1idrfljkzv8j0f6xc3821uv3.png)
Since 0.50 lies in the interval hence we fail to reject
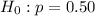
Thus, the method does not appear to be effective.