Answer:
The mean of the sampling proportions is 0.62.
The standard error for the distribution of sampling proportions is 0.069.
Explanation:
The proportion of graduates from public universities that had student loans is p=0.62.
The sampling distribution of the proportions, if the sample are of size n=50, has the following parameters:
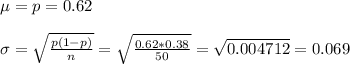
The mean of the sampling proportions is 0.62.
The standard error for the distribution of sampling proportions is 0.069.