Question

An open-top box is to be made from a 70-centimeter by 96-centimeter piece of plastic by removing a square from each corner of the plastic and folding up the flaps on each side. What size square should be cut out of each corner to get a box with the maximum volume

Answer 1

Therefore, the length of the square is
`(40)/(3)` cm which should be cut out of each corner to get a box with the maximum volume.

Explanation:

Given that, an open box is made from a plastic with dimension 70 cm by 96 cm by removing a square from each corner of the plastic.

Consider, x be the length of the side of the square.

After cutting the corner, the length of the plastic is = (70-2x) cm

The width of the plastic is=(96-2x) cm.

Now the length of the open box = (70-2x) cm

The width of the open box = (96-2x) cm.

Then x will be the height of the open box.

The volume of the box is

V=Length×width×height

=[(70-2x) (96-2x) x] cm³

=(6720x -192x²-140x²+4x³) cm³

=(4x³-332x²+6720x) cm³

∴V=4x³-332x²+6720x

Differentiating with respect to x

V'= 12x²-664x+6720

Again differentiating with respect to x

V''= 24x -664

To find maximum volume, we set V' =0

12x²-664x+6720=0

⇒4(3x²-166x+1680)=0

⇒3x²-166x+1680=0

⇒3x²-126x-40x+1680=0

⇒3x(x-42)-40(x-42)=0

⇒(x-42)(3x-40)=0

`\Rightarrow x = 42,(40)/(3)`

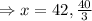
Now

`V''|_(x=42)=(24* 42)-664=334>0`

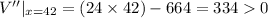
And

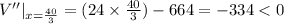`V''|_{x=(40)/(3)}=(24* (40)/(3))-664=-334<0` At
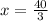`x=(40)/(3)` , V has maximum value.

Therefore, the length of the square is
`(40)/(3)` cm which should be cut out of each corner to get a box with the maximum volume.